Statistical Design of Experiments
Statistical Design of Experiments
Statistical Design of Experiments
Statistical Design of Experiments
One -Way ANOVA
One -Way ANOVA
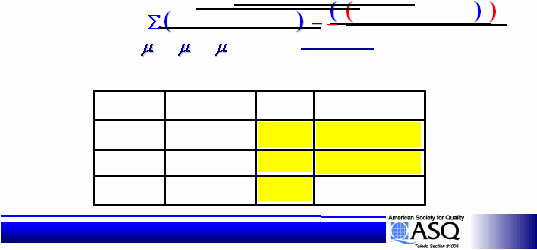
The Formulas:
The Formulas:
Total SS =
Total SS =
ObsObs
TotalTotal
ObservationsObservations
TotalTotal
Observation
each
#
2
2
2
2
SS
SS
Input
Input
Factor
Factor
= SS
= SS
#
2
2
The sum of each
input Factor level
total squared
# Obs in each input
factor level
SS
Error
Error
= Total SS
= Total SS
Total SS
-
-
SS
SS
Input
Input
Factor
Factor
Source
d.f.
SS
MS ~ Variance
Input
Factor
Error
TOTAL
# levels -
1
d.f.
Tot
-
d.f.
In Fac
(# Obs-1)
SS
In Fac
SSE
Total SS
SS
In Fac
/ d.f.
In Fac
SSE / d.f.
Error
SS
In
Fac
SSE
Total SS
SS
In
Fac
/ d.f.
In Fac
SSE / d.f.
Error
MSMSE =
MS
Input
Input
Factor
Factor
=
=
SS
SS
Input
Input
Factor
Factor
# input Factor levels-1
# input Factor levels-1
1
Where MS = Input factor mean sum of squares (variance)
Where MS = Input factor mean sum of squares (variance)
MSE =
SS
SS
Error
Error
n -
n -
# input factors
# input factors
Where MSE = Error mean sum of squares (variance)
Where MSE = Error mean sum of squares (variance)
To reject the null hypothesis, the F statistic must be
To reject the null hypothesis, the F statistic must be
greater than the critical value of F found in an F Table
greater than the critical value of F found in an F Table
H
H
O
O
:
:
1
1
=
=
2
2
=
=
3
3
...
...
F =
F =
MSE
MSE
MS
MS
In
In
Fac
Fac
> F
> F
Table
Table